ASCE 7 Seismic Design – Part 1

We are going to break down and review seismic design in regards to ASCE7-05. We are going to cover the basics and some commentary. Hopefully I will be able to elaborate sometime in the future and include some discussion.

Based ASCE7-05

1)        Exceptions

• a)        Detached 1 and 2 family dwellings with a Ss<0.4 and SDC (Seismic Design Category) = A, B or C.
• b)       Detached 1 and 2 family dwellings (not included above), wood framed, less than 2 stories, and designed in accordance with the IRC.
• c)        Agricultural buildings
• d)       Structures not included in ASCE7 such as bridges, transmission towers, nuclear, and buried structures.

2)       Existing Structures – Alterations and additions

• a)        See appendix 11B .
• b)       To summarize – You have three options
  • i)        Design the addition/alteration separately
  • ii)       If the alteration/addition does NOT increase the seismic force or reduce the strength of the existing structural member(s) by more than 10% than you do NOT need to upgrade the existing member(s) to meet the current standard.
  • Upgrade the existing to meet the current standard (code)

Seismic Loads

First determine how the earth movement will influence the building and what acceleration should be used to model this movement.

1. Determine Ss and S1 from the 0.2 and 1.0s spectral response accelerations shown on Figs. 22-1 through 22-14.
2. Determine the Site Class based on soil properties. (See chapter 20 for more info on determining site classes). The site class shall be A,B,C,D,E,F based on the geotechnical report or local AHJ. If there has not been a site class provided site class D may be assumed (unless otherwise specified by AHJ).
3. Determine the appropriate site coefficient, Fa based on the short period, Ss, and Fv based on the 1sec period, S1, and the site class (Table 11.4-1 and 11.4-2).
   • Evaluate the Maximum Considered Earthquake (MCE) spectral response acceleration for short periods and 1 sec periods.
     • Sms=Fa*Ss
     • Sm1=FvS1
4. Design Spectral Acceleration Parameters (these are the values used in design).
   • Sds=2/3*Sms (short period)
   • Sd1=2/3*Sm1 (1 s period)
5. Determine Occupancy Category from Table 1-1
6. Determine Importance Factor from Table 11.5-1
7. Determine Seismic Design Category (SDC) A,B,C ,D, E or F. (E is reserved for S1>0.75 and F is reserved for Occupancy Category IV w/ S1>0.75) based occupancy category and period response acceleration parameter.
   • Table 11.6-1 SDC based on Sds
   • Table 11.6-2 SDC based Sd1
   • Use the most severe case. It is permitted to use Table 11.6-1 if S1 < 0.75 and all of the following apply:
   1. Unless Ta<0.8*Ts ,
   2. In each orthogonal direction the fundamental period of the structure used to calculate story drift is less than Ts
   3. Eq 12.8-2 is used to calc Cs (Cs=Sds/(R*I)) seismic response coefficient.
   4. Diaphragms are rigid or flexible diaphragms with vertical elements of the LFRS do not exceed 40′ spacing.
8. Some quick notes for designing building in SDC A:
   1. For SDC A the force at each level may be determined by Fx=0.01*Wx
      • Essentially 1% of the weight is used as horizontal force.
   2. Load path connections for a smaller portion of the structure connected to the ‘main’ structure shall be designed using 0.05*(Rdl+Rll) (Dead load and live load reaction) of the smaller portions weight. Connections shall also be designed for 0.05*W. Unless the lateral system cannot provide for this force than the maximum force the the lateral system can provide should be used.
   3. Anchorage to concrete or masonry walls. The minimum connection to the diaphragm shall be 280 plf (strength level). This should be substituted for E in the load combinations.

Second – How will the building respond and what forces should be used based on the ground acceleration (found previously) and how the building responds.

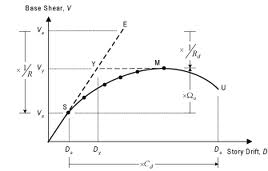
1. Select a lateral force resisting system. For each system Table 12.2-1 lists the following: Response Modification Factor (R), System Overstrength factor (Ω Omega) and Deflection Amplification Factor (Cd).
   • For a more indepth explanation see here:http://www.structuremag.org/article.aspx?articleID=756
   • R: The response modification factor reduces the seismic load to strength level design forces. Essentially this accounts for the ductility of the structure. Systems that can dissipate energy through a ductile response have higher R factor. These systems may require a higher level of design and detailing for certain SDC’s.
   • Ω Omega: The Overstrength factor increases the required seismic forces and is applied in specific cases or in the design of certain parts of the structure. Ω0 is intended to reflect the upper bound lateral strength of the structure and estimates the maximum forces in elements that are to remain non-yielding during the design basis ground motion. In summary, R reduces the required seismic forces realizing the some yielding of the structure will help dissipate energy. To force a more ductile response some ‘brittle’ members are designed to resist higher forces so that they stay in the elastic range during the seismic event.
   • (Cd) Deflection Amplification Factor: Realizing that the structure is intended to yield (ductile response) deflection will be greater than that found from an elastic analysis. Cd amplifies the deflection of the structure based on an elastic analysis.

   • 

     Response Factor, Deflection Amplification Factor and Overstrength Factor

2. Different systems may be used in the same structure. If the systems are in orthogonal directions the R, Omega, Cd shall be applied to each system. Systems used in combination to resist lateral forces in the same direction are referred to as dual systems. Some dual systems are listed in Table 12.2-1. For other systems the more stringent system limitation shall apply.
   • If R, C and Ω vary over the height of the structure; the story below shall meet the most stringent of the stories above (avoid weak story) for systems in the same direction.
     • There are multiple exceptions see (12.2.3.1)
   • If  R, C and Ω vary within the same story (Horizontal Combinations). R shall be the lowest of the different systems for that story. R may vary for different lines of LFRS if the building category is 1 or 2, two stories or less and the use of flexible diagrams. However the diaphragm shall use the lowest R value. Cd and Omega  in the direction under consideration under consideration at any story shall not be less than the largest value of this factor for the R factor used in the same direction being considered.
   • Further restrictions and direction is given in 12.2.5 for specific system requirements.
3. Irregularities – Irregularities are covered in chapter 12.3. They are specific to certain geometries and mass distributions.
   • Vertical Irregularities – Differences from story to story including – Variable stiffness, variable weight distribution, offset of vertical elements.
   • Horizontal Irregularities – Reentrant corners, torsional, discontinuous diaphragms, non parallel systems.
4. Redundancy Factor, ρ – equal to 1 for the following:
   • Structures assigned to SDC B or C
   • Calculating drift and P-Delta effects.
   • Design of nonbuilding structures
   • Design of collector elements, splices and connections when using the overstrength factor.
   • Diaphragm loads using Eq 12.10-1
   • Structures with damping  systems (Section 18).
5. Redundancy Factor, ρ – equal to 1.3 for SDC D,E and F. Unless the exceptions of 12.3.4.2 are met and comply with table 12.3-3.
6. Diaphragm Flexibility – Rigid, Flexible and Semi-Rigid. All diaphragms are semi-rigid, meaning that load is distributed to from the diaphragm to the vertical elements depends on the stiffness of the diaphragm and stiffness of the vertical elements.
   1. Rigid – When concrete is used, span-to-width is <3 and no horizontal irregularities.
   2. Flexible – selective combinations of materials used for vertical elements and diaphragms. In general if the maximum diaphragm deflection is  less than 2 x the average drift of the vertical element.
7. Continuous Load Path – Any smaller portion of the structure shall be connected to the remainder of the structure and designed to transmit 0.133*Sds*W or 0.05*W of the smaller portion.
8. Connection to supports  – a minimum of 0.05*(Wdl+WLL) of the beam/girder/truss reaction.

Next we will consider how to apply the loads including load combinations, magnitude, direction and modeling.